|
Spacecraft propulsion is any method used to change the velocity of
spacecraft
and artificial
satellites.
There are many different methods. Each method has drawbacks and advantages, and
spacecraft propulsion is an active area of research. However, most spacecraft
today are propelled by exhausting a gas from the back/rear of the vehicle at
very high speed through a
supersonic de Laval nozzle. This sort of
engine is
called a
rocket engine.
All current spacecraft use chemical rockets (bipropellant
or
solid-fuel) for launch, though some (such as the
Pegasus rocket and
SpaceShipOne) have used
air-breathing engines on their
first stage. Most satellites have simple reliable chemical thrusters (often
monopropellant rockets) or
resistojet rockets for
orbital station-keeping and some use
momentum wheels for
attitude control. Soviet bloc satellites have used
electric propulsion for decades, and newer Western geo-orbiting spacecraft
are starting to use them for north-south stationkeeping. Interplanetary vehicles
mostly use chemical rockets as well, although a few have experimentally used
ion
thrusters (a form of electric propulsion) to great success.
The necessity for propulsion system
Artificial satellites must be
launched into
orbit, and once there they must be placed in their nominal orbit. Once in
the desired orbit, they often need some form of
attitude control so that they are correctly pointed with respect to the
Earth, the
Sun, and possibly
some
astronomical object of interest.
They are also subject to
drag from the thin
atmosphere, so that to stay in orbit for a long period of time some form of
propulsion is occasionally necessary to make small corrections (orbital
stationkeeping).
Many satellites need to be moved from one orbit to another from time to time,
and this also requires propulsion.
When a satellite has exhausted its ability to adjust its orbit, its useful life
is over.
Spacecraft designed to travel further also need propulsion methods. They need
to be launched out of the Earth's atmosphere just as satellites do. Once there,
they need to leave orbit and move around.
For
interplanetary travel, a spacecraft must use its engines to leave Earth
orbit. Once it has done so, it must somehow make its way to its destination.
Current interplanetary spacecraft do this with a series of short-term trajectory
adjustments.
In between these adjustments, the spacecraft simply
falls freely along its orbit. The simplest fuel-efficient means to move from
one circular orbit to another is with a
Hohmann transfer orbit: the spacecraft begins in a roughly circular orbit
around the Sun. A short period of
thrust in the
direction of motion accelerates or decelerates the spacecraft into an elliptical
orbit around the Sun which is tangential to its previous orbit and also to the
orbit of its destination. The spacecraft falls freely along this elliptical
orbit until it reaches its destination, where another short period of thrust
accelerates or decelerates it to match the orbit of its destination.
Special methods such as
aerobraking are sometimes used for this final orbital adjustment.
Some spacecraft propulsion methods such as
solar sails
provide very low but inexhaustible thrust;
an interplanetary vehicle using one of these methods would follow a rather
different trajectory, either constantly thrusting against its direction of
motion in order to decrease its distance from the Sun or constantly thrusting
along its direction of motion to increase its distance from the Sun.
Spacecraft for
interstellar travel also need propulsion methods. No such spacecraft has yet
been built, but many designs have been discussed. Since interstellar distances
are very great, a tremendous velocity is needed to get a spacecraft to its
destination in a reasonable amount of time. Acquiring such a velocity on launch
and getting rid of it on arrival will be a formidable challenge for spacecraft
designers.
Effectiveness of propulsion systems
When in space, the purpose of a propulsion system is to change the velocity,
or v, of a spacecraft. Since this is more difficult for more massive
spacecraft, designers generally discuss
momentum,
mv. The amount of change in momentum is called
impulse.
So the goal of a propulsion method in space is to create an impulse.
When launching a spacecraft from the Earth, a propulsion method must overcome
a higher
gravitational pull to provide a net positive acceleration.
In orbit, any additional impulse, even very tiny, will result in a change in the
orbit path.
The rate of change of velocity is called
acceleration, and the rate of change of momentum is called
force. To reach a
given velocity, one can apply a small acceleration over a long period of time,
or one can apply a large acceleration over a short time. Similarly, one can
achieve a given impulse with a large force over a short time or a small force
over a long time. This means that for maneuvering in space, a propulsion method
that produces tiny accelerations but runs for a long time can produce the same
impulse as a propulsion method that produces large accelerations for a short
time. When launching from a planet, tiny accelerations cannot overcome the
planet's gravitational pull and so cannot be used.
The Earth's surface is situated fairly deep in a
gravity well and it takes a velocity of 11.2 kilometers/second (escape
velocity) or more to escape from it. As human beings evolved in a
gravitational field of 1g (9.8 m/s�), an ideal propulsion system would be one
that provides a continuous acceleration of 1g (though human bodies can
tolerate much larger accelerations over short periods). The occupants of a
rocket or spaceship having such a propulsion system would be free from all the
ill effects of
free fall, such as nausea, muscular weakness, reduced sense of taste, or
leaching of calcium from their bones.
The law of
conservation of momentum means that in order for a propulsion method to
change the momentum of a space craft it must change the momentum of something
else as well. A few designs take advantage of things like magnetic fields or
light pressure in order to change the spacecraft's momentum, but in free space
the rocket must bring along some mass to accelerate away in order to push itself
forward. Such mass is called reaction mass.
In order for a rocket to work, it needs two things: reaction mass and energy.
The impulse provided by launching a particle of reaction mass having mass m
at velocity v is mv. But this particle has kinetic energy mv�/2,
which must come from somewhere. In a conventional
solid,
liquid, or
hybrid rocket, the fuel is burned, providing the energy, and the reaction
products are allowed to flow out the back, providing the reaction mass. In an
ion
thruster, electricity is used to accelerate ions out the back. Here some
other source must provide the electrical energy (perhaps a
solar panel or a
nuclear reactor), while the ions provide the reaction mass.
When discussing the efficiency of a propulsion system, designers often focus
on effectively using the reaction mass. Reaction mass must be carried along with
the rocket and is irretrievably consumed when used. One way of measuring the
amount of impulse that can be obtained from a fixed amount of reaction mass is
the
specific impulse, the impulse per unit weight-on-Earth (typically designated
by Isp). The unit for this
value is seconds. Since the weight on Earth of the reaction mass is often
unimportant when discussing vehicles in space, specific impulse can also be
discussed in terms of impulse per unit mass. This alternate form of specific
impulse uses the same units as velocity (e.g. m/s), and in fact it is equal to
the effective exhaust velocity of the engine (typically designated
ve). Confusingly, both
values are sometimes called specific impulse. The two values differ by a factor
of
gn, the standard acceleration due to gravity 9.80665 m/s�
(Ispgn = ve).
A rocket with a high exhaust velocity can achieve the same impulse with less
reaction mass. However, the energy required for that impulse is proportional to
the square of the exhaust velocity, so that more mass-efficient engines require
much more energy, and are typically less energy efficient. This is a problem if
the engine is to provide a large amount of thrust. To generate a large amount of
impulse per second, it must use a large amount of energy per second. So highly
(mass) efficient engines require enormous amounts of energy per second to
produce high thrusts. As a result, most high-efficiency engine designs also
provide very low thrust.
Delta-v and propellant use
Burning the entire usable propellant of a spacecraft through the engines in a
straight line in free space would produce a net velocity change to the vehicle;
this number is termed 'delta-v'
(Δv).
If the exhaust velocity is constant then the total Δv
of a vehicle can be calculated using the rocket equation, where M is the
mass of propellant, P is the mass of the payload (including the rocket
structure), and ve is the
velocity of the rocket exhaust. This is known as the
Tsiolkovsky rocket equation:
-
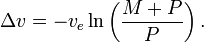
For historical reasons, as discussed above, ve
is sometimes written as
- ve = Ispgo
where Isp is the
specific impulse of the rocket, measured in seconds, and
go is the
gravitational acceleration at sea level.
For a high delta-v mission, the majority of the spacecraft's mass needs to be
reaction mass. Since a rocket must carry all of its reaction mass, most of the
initially-expended reaction mass goes towards accelerating reaction mass rather
than payload. If the rocket has a payload of mass P, the spacecraft needs
to change its velocity by Δv, and the rocket
engine has exhaust velocity ve, then the mass M of
reaction mass which is needed can be calculated using the rocket equation and
the formula for Isp:
-
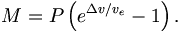
For Δv much smaller than ve,
this equation is roughly
linear, and
little reaction mass is needed. If Δv is
comparable to ve, then there needs to be about twice as much
fuel as combined payload and structure (which includes engines, fuel tanks, and
so on). Beyond this, the growth is exponential; speeds much higher than the
exhaust velocity require very high ratios of fuel mass to payload and structural
mass.
For a mission, for example, when launching from or landing on a planet, the
effects of gravitational attraction and any atmospheric drag must be overcome by
using fuel. It is typical to combine the effects of these and other effects into
an effective mission
delta-v. For
example a launch mission to low Earth orbit requires about 9.3-10 km/s delta-v.
These mission delta-vs are typically numerically integrated on a computer.
|

