- Residue Curve Maps
The most simple form of distillation, called simple
distillation, is a process in which a muticomponent liquid mixture is slowly
boiled in an open pot and the vapors are continuously removed as they form. At
any instant in time the vapor is in equilibrium with the liquid remaining on the
still. Because the vapor is always richer in the more volatile components than
the liquid, the liquid composition changes continuously with time, becoming more
and more concentrated in the least volatile species. A simple distillation
residue curve is a graph showing how the composition of the liquid residue
curves on the pot changes over time. A residue curve map is a collection
of the liquid residue curves originating from different initial compositions.
Residue curve maps contain the same information as phase diagrams, but represent
this information in a way that is more useful for understanding how to
synthesize a distillation sequence to separate a mixture.
All of the residue curves originate at the light (lowest
boiling) pure component in a region, move towards the intermediate boiling
component, and end at the heavy (highest boiling) pure component in the same
region. The lowest temperature nodes are termed as unstable nodes (UN),
as all trajectories leave from them; while the highest temperature points in the
region are termed stable nodes (SN), as all trajectories ultimately reach
them. The point that the trajectories approach from one direction and end in a
different direction (as always is the point of intermediate boiling component)
are termed saddle point (S). Residue curve that divide the composition
space into different distillation regions are called distillation boundaries. A
better understanding of the residue curve map may be view in Fig. 1.
Notice that the trajectories move from the lowest temperature component towards
the highest.
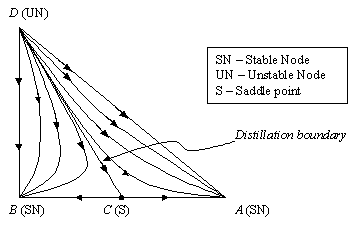
Fig. 1 Residue curve
map for a ternary mixture with a distillation boundary running
from pure component D to the binary azeotrope C.
Residue curve maps would be of limited usefulness if they could only be
generated experimentally. Fortunately that is not the case. Using various
references, the simple distillation process can be described by the set of
equations:

Research studies have also been done to determine the
relationship between the number of nodes (stable and unstable) and saddle points
one can have in a legitimately drawn ternary residue plot. The equation is based
on topological arguments. One form for this equation is:
4(N3 - S3) + 2(N2 - S2)
+ (N1 - S1) = 1
Where:
Ni = number of nodes (stable and unstable) involving i
species
Si = number of saddles involving i
species
Many different residue curve maps are possible when
azeotropes are present. Ternary mixtures containing only one azeotrope may
exhibit six possible residue curve maps that differ by the binary pair forming
the azeotrope and by whether the azeotrope is minimum or maximum boiling.
Even though the simple distillation process has no practical
use as a method for separating mixtures, simple distillation residue curve maps
have extremely useful applications, such as:
- Testing of the consistency of experimental azeotropic data;
- Predict the order and content of the cuts in batch distillation;
- In distillation, to check whether the given mixture is separable by
distillation, identification of the entrainers / solvents, prediction of
attainable product compositions, qualitative prediction of composition
profile shape, and synthesis of the corresponding distillation column.
By identifying the limiting separation achievable by
distillation, residue curve maps are also useful in synthesizing separation
sequences combining distillation with other methods.
|

