Quartz crystals and the quartz crystal resonator
- overview, information or tutorial about the basics of quartz crystals, and
they may be used as a quartz crystal resonator in a radio frequency oscillator
or filter.
Quartz crystals are widely used in today's electronics
circuits as high quality tuned circuits or resonators. Despite their high
performance quartz crystals are cheap to produce and they find many uses in
applications from oscillator clock circuits in microprocessor boards, the timing
element in digital watches as well as their more traditional applications in
radio frequency applications where they may be used as the resonators in highly
stable quartz crysal oscillators of high performance crystal filters.
As the name implies quartz crystal resonators are made from
quartz, a naturally occurring form of silicon, although most of that used for
electronics applications is manufactured synthetically these days. The
components rely on the remarkable properties of quartz for their operation. When
placed into an electronic circuit a crystal acts as a tuned circuit. However it
has an exceptionally high Q. Ordinary LC tuned circuits may exhibit values of a
few hundred if carefully designed and constructed, but quartz crystals exhibit
values of up to 100 000. Apart from their Q, crystals also have a number of
other advantages. Their stability is remarkably good with respect to temperature
and time. In fact most crystals will have these figures specified and they might
typically be �5 ppm (parts per million) per year for the ageing and �30 ppm over
a temperature range of 0 to 60 degrees Celsius.
How quartz crystal resonators work
A quartz crystal resonator depends on the piezo-electric
effect to work. This effect converts a mechanical stress in a crystal to a
voltage and vice versa. In this way the piezo-electric effect converts the
electrical impulses to mechanical stress which is subject to the very high Q
mechanical resonances of the crystal, and this is in turn linked back into the
electrical circuit.
The quartz crystal can vibrate in several different ways, and
this means that it has several resonances, all on different frequencies.
Fortunately the way in which the quartz crystal blank is cut from the original
crystal itself can very significantly reduce this. In fact the angle of the
faces relative to the original crystal axes determines many of its properties
from the way it vibrates to its activity, Q, and its temperature co-efficient.
There are three main ways in which a crystal can vibrate: longitudinal mode, low
frequency face shear mode, and high frequency shear. A cut known as the AT cut
used for most crystals used in traditional radio and electronics circuits uses
the high frequency shear mode.
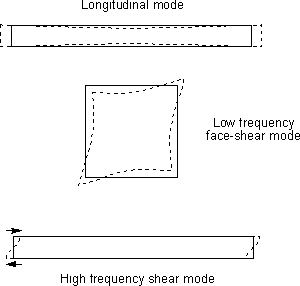
Vibrational modes of a quartz crystal resonator
(For the sake of clarity, the movements have been greatly exaggerated)
Equivalent circuit of a quartz crystal resonator
To analyse the electrical response of a quartz crystal
resonator, it is very often useful to depict it as the equivalent electrical
components that would be needed to replace it. This equivalent circuit is can
then be used to analyse its response and predict its performance. The basic
equivalent circuit of a crystal is shown below. In this circuit C1 represents
the capacitance between the electrodes. L, C, and R represent the vibrational
characteristics of the crystal. The inductance results from the mass of the
material, C from the compliance, and R arises from the losses of which the
greatest contributor is frictional losses.
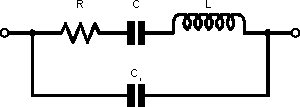
The equivalent circuit of a quartz crystal resonator
Looking at this circuit it can be seen that there are two
ways in which the circuit can resonate. One is from the resonance of L and C
which provides a series resonance, giving a very low value of impedance at
resonance. This is determined by the value of the resistance R. In this mode the
external circuit has very little effect on the crystal resonance.
The other is a parallel resonance providing a very high
impedance. This occurs when the combination of L, and C has an inductive
reactance that equals C1 together with any value of capacitance provided by the
external circuit. It is for this reason that crystals designed to operate in
this mode have a value of load capacitance specified. This value of capacitance
must be provided by the external circuit if the crystal is to operate at its
specified frequency.
Quartz crystal resonators can operate in either mode, and in
fact the difference between the parallel and series resonant frequencies is
quite small. Typically they are only about 1% apart. Of the two modes, the
parallel mode is more commonly used, but either may be found. Oscillator
circuits for using the different modes are naturally different, as one
oscillates when the crystal reaches its maximum impedance whilst the other
operates when the crystal reaches its minimum impedance.
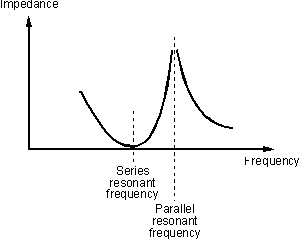
Impedance characteristics of a quartz crystal resonator
Apart from their use in oscillators, quartz crystals find
uses in filters. Here they offer levels of performance that cannot be achieved
by other forms of filter. Often several crystals may be used in one filter to
provide the correct shape.
How quartz crystal resonators are made
The individual quartz crystal resonators are manufactured
from large man-made crystals that are generally several inches long. They are
around two inches in diameter and have a hexagonal cross section. The individual
quartz crystals are cut from the large crystal using diamond wheels. These are
required in view of the hardness of the material. The angle of the cut to the
axes of the original crystal is determined very accurately to ensure the final
crystal has the right properties. The blanks that are created from the cutting
process are in the form of discs, often about the size of a small coin, although
this varies according to their final frequency of operation. Once the blanks
have been cut they are lapped using a very fine paste to bring them to nearly
the right size. The lapping paste normally consists of very fine silicon carbide
or aluminium oxide. The final stage of preparation usually involves chemical
etching, because this process enables the required very fine finish to be
obtained.
The next stage in manufacture involves mounting the quartz
crystal. Silver or gold contacts are chemically deposited onto both sides of the
blank. The amount of metal that is used in this process can be used to trim the
operating frequency of the crystal to its final value. Finally the crystal is
mounted into its can or glass envelope. This is either evacuated or filled with
an inert gas to minimise ageing.
Specifying quartz crystal resonators
When choosing a quartz crystal resonator there are many
parameters that need to be selected. Many are fairly simple like the tolerance
figures. However a few of the others need a little extra explanation. One is the
type of resonance. Like any tuned circuit a crystal can have a parallel or
series form of resonance as shown. This will have to be specified. If the
crystal is to have a parallel resonance then a load capacitance will have to be
chosen. This is required because any capacitance across the crystal will alter
its resonance slightly. Typically this might be 30 pF, but it will be dependent
upon the circuit to be used. Also the tolerance required must be specified. The
closer the tolerance, the more expensive the crystal will be, so it is wise not
to over-specify the item.
Quartz crystal resonator overview
Quartz crystal resonators are widely used within the
electronics industry. They can be sued in quartz crystal oscillators and crystal
filters where they provide exceptionally high levels of performance. In addition
to this, low cost elements with lower tolerance specifications are widely used
in crystal oscillators for microprocessor board clocks where they are used as
cheap resonator elements. Whatever its use a quartz crystal resonator provides
an exceptionally high level of performance for the cost of its production.
|

