Error Propagation

Error Propagation

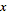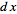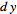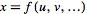Given a formula with an absolute error in of , the absolute error is . The relative error is . If , then

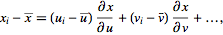(1)

where 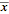denotes the mean, so the sample variance is given by

			(2)
			(3)

The definitions of variance and covariance then give

			(4)
			(5)
			(6)

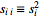(where ), so

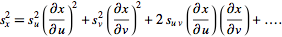(7)

If 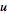and 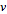are uncorrelated, then 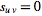so

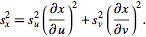(8)

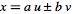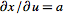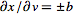Now consider addition of quantities with errors. For , and , so

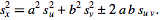(9)

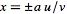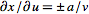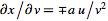For division of quantities with , and , so

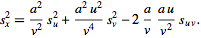(10)

Dividing through by 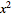and rearranging then gives

			(11)
			(12)
			(13)

For exponentiation of quantities with

(14)

and

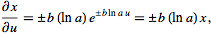(15)

so

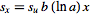(16)

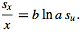(17)

If 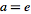, then

(18)

For logarithms of quantities with 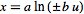, , so

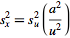(19)

(20)

For multiplication with 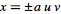, 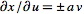and , so

(21)

			(22)
			(23)

For powers, with 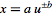, , 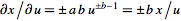so

(24)