Circuit Analysis Technique

Circuit Analysis Techniques

Fundamentals

Ohm's Law states the voltage across a resistor, R (or impedance, Z) is directly proportional to the current passing through it (the resistance/impedance is the proportionality constant)

Kirchhoff's Voltage Law (KVL): the algebraic sum of the voltages around any loop of N elements is zero (like pressure drops through a closed pipe loop)

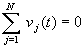
Kirchhoff's Current Law (KCL): the algebraic sum of the currents entering any node is zero, i.e., sum of currents entering equals sum of currents leaving (like mass flow at a junction in a pipe)

Nodal Analysis

Nodal analysis is generally best in the case of several voltage sources. In nodal analysis, the variables (unknowns) are the "node voltages."

Nodal Analysis Procedure:

1. Label the N node voltages. The node voltages are defined positive with respect to a common point (i.e., the reference node) in the circuit generally designated as the ground (V = 0).
2. Apply KCL at each node in terms of node voltages.
   1. Use KCL to write a current balance at N-1 of the N nodes of the circuit using assumed current directions, as necessary. This will create N-1 linearly independent equations.
   2. Take advantage of supernodes, which create constraint equations. For circuits containing independent voltage sources, a supernode is generally used when two nodes of interest are separated by a voltage source instead of a resistor or current source. Since the current (i) is unknown through the voltage source, this extra constraint equation is needed.
   3. Compute the currents based on voltage differences between nodes. Each resistive element in the circuit is connected between two nodes; the current in this branch is obtained via Ohm's Law where V_m is the positive side and current flows from node m to n (that is, I is m --> n).

   

3. Determine the unknown node voltages; that is, solve the N-1 simultaneous equations for the unknowns, for example using Gaussian elimination or matrix solution methods.

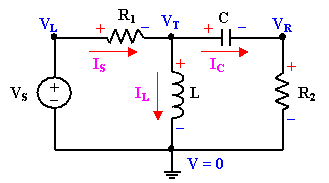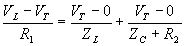
Label the nodal voltages. Apply KCL. KCL at top node gives IS = IL + IC Supernode constraint eq. of VL = VS Solve for VT for instance.

Loop or Mesh Analysis

Mesh (loop) analysis is generally best in the case of several current sources. In loop analysis, the unknowns are the loop currents. Mesh analysis means that we choose loops that have no loops inside them.

Loop Analysis Procedure:

1. Label each of the loop/mesh currents.
2. Apply KVL to loops/meshes to form equations with current variables.
   1. For N independent loops, we may write N total equations using KVL around each loop. Loop currents are those currents flowing in a loop; they are used to define branch currents.
   2. Current sources provide constraint equations.
3. Solve the equations to determine the user defined loop currents.

Label mesh currents. Apply KVL. Left loop KVL: VS = R1I1 + R2(I1-I2) Constraint equation I2 = -IS. Solve for I1 and I2. Note: Branch current from mesh currents: IM = I1 - I2

Superposition

In any linear circuit containing multiple independent sources, the current or voltage at any point in the network may be calculated as the algebraic sum of the individual contributions of each source acting alone.

Procedure:

1. For each independent voltage and current source (repeat the following):
   1. Replace the other independent voltage sources with a short circuit (i.e., v = 0).
   2. Replace the other independent current sources with an open circuit (i.e., i = 0).
   Note: Dependent sources are not changed!
   Calculate the contribution of this particular voltage or current source to the desired output parameter.
2. Algebraically sum the individual contributions (current and/or voltage) from each independent source.

Source Transformation

An ac voltage source V in series with an impedance Z can be replaced with an ac current source of value I=V/Z in parallel with the impedance Z.
An ac current source I in parallel with an impedance Z can be replaced with an ac voltage source of value V=IZ in series with the impedance Z.

Likewise, a dc voltage source V in series with a resistor R can be replaced with a dc current source of value i = v/R in parallel with the resistor R; and vice versa.

Th�venin's and Norton's Theorems

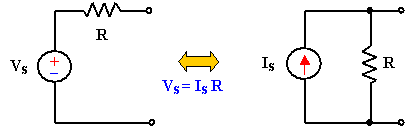
Th�venin's Theorem states that we can replace entire network, exclusive of the load, by an equivalent circuit that contains only an independent voltage source in series with an impedance (resistance) such that the current-voltage relationship at the load is unchanged.

Norton's Thereom is identical to Th�venin's Theorem except that the equivalent circuit is an independent current source in parallel with an impedance (resistor). Hence, the Norton equivalent circuit is a source transformation of the Th�venin equivalent circuit.

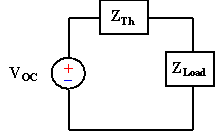
Th�venin Equivalent Circuit	Norton Equivalent Circuit

Procedure:

1. Pick a good breaking point in the circuit (cannot split a dependent source and its control variable).
2. Thevenin: Compute the open circuit voltage, V_OC.
   Norton: Compute the short circuit current, I_SC.
3. Compute the Thevenin equivalent resistance, R_Th (or impedance, Z_Th).
   1. If there are only independent sources, then short circuit all the voltage sources and open circuit the current sources (just like superposition).
   2. If there are only dependent sources, then must use a test voltage or current source in order to calculate R_Th= v_Test/i_Test (or Z_Th=V_Test/I_Test).
   3. If there are both independent and dependent sources, then compute R_Th (or Z_Th) from R_Th= v_OC/i_SC (or Z_Th=V_OC/I_SC).
4. Replace circuit with Thevenin/Norton equivalent.
   Thevenin: V_OC in series with R_Th (or Z_Th).
   Norton: I_SC in parallel with R_Th (or Z_Th).
Note: for 3(b) the equivalent network is merely R_Th (or Z_Th), that is, no current or voltage sources.