The logarithm of a complex number.
Definition 4.4.1
,
|
where
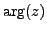
is defined up to an additive multiple of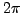 
.
We denote
Log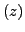
the principal value of 
,
i.e. the value corresponding to the principal value
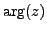of
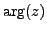(recall that
).
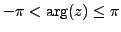Proposition 4.4.5
The logarithmic function is analytic on its domain.
For a proof, use Cauchy-Riemann equations (v.s. 3).
Example 4.4.7
The proof is simple: let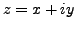 
, where
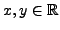. Then we have:
and
Proposition 4.8 means that the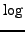 
function is not exactly the inverse of the
complex exponential function in basis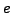 
.
|
