System of Partial Differential Equations: How to Solve Maxwell's Equations Using Linear Algebra

System of Partial Differential Equations

System of Partial Differential Equations: How to Solve Maxwell's Equations Using Linear Algebra

The theme of the ensuing development is linear algebra, but the subject is an overdetermined system of partial differential equations, namely, the Maxwell field equations. The objective is to solve them via the method of eigenvectors and eigenvalues. The benefit is that the task of solving the Maxwell system of p.d. equations is reduced to solving a single inhomogeneous scalar equation⁶⁴

$\displaystyle \left( \partial^2_x+\partial^2_y+\partial^2_z -\partial^2_t \right) =-4\pi S(t,\vec x)~,$

where 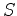is a time and space dependent source. The impatient reader will find that once this master equation, or its manifestation in another coordinate system, has been solved, the electric and magnetic fields are entirely determined as in Tables [*] -6.9.

The starting point of the development is Maxwell's equations. There is the set of four functions, the density of charge

$\displaystyle \rho$	$\displaystyle =\rho(\vec x,t) \quad \left[\frac{\textrm{(charge)}}{\textrm{(volume)}}\right]$	(637)
and the charge flux
$\displaystyle \vec J$	$\displaystyle = \vec J(\vec x,t) \quad \left[\frac{\textrm{(charge)}}{\textrm{(time)(area)}}\right],$	(638)

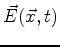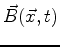which are usually given. These space and time dependent charge distributions give rise to electric and magnetic fields, $\vec E(\vec x,t)$ and $\vec B(\vec x,t)$ . The relationship is captured by means of Maxwell's gift to twentieth century science and technology,

$\displaystyle \nabla \cdot \vec B$	$\displaystyle =0 \quad ~~ \textrm{(\lq\lq No magnetic monopoles'')}$	(639)

$\displaystyle \nabla \times \vec E +\frac{\partial \vec B}{\partial t}$	$\displaystyle =0 \quad ~~ \textrm{(\lq\lq Faraday's law'')}$	(640)
and
$\displaystyle \nabla \cdot \vec E$	$\displaystyle =4\pi \rho \quad \textrm{(\lq\lq Gauss' law'')}$	(641)
$\displaystyle \nabla \times \vec B -\frac{\partial \vec E}{\partial t}$	$\displaystyle =4\pi \vec J\quad \textrm{(\lq\lq Ampere's law'')}~,$	(642)

Maxwell's field equations⁶⁵.

Exercise 62.1 (Charge Flux-Density of an Isolated Charge)
Microscopic observations show that charged matter is composed of discrete point charges. On the other hand, macroscopic observations show that charged matter is the carrier of an electric fluid which is continuous. Dirac delta functions provide the means to grasp both attributes from a single perspective. This fact is highlighted by the following problem.

Consider the current-charge density due to an isolated moving charge,

$\displaystyle \vec J(x,y,z,t)$	$\displaystyle =q\int_{-\infty}^\infty \frac{d\vec X(\tau)}{d\tau} \delta(x-X(\tau))\delta(y-Y(\tau)) \delta(z-Z(\tau))\delta(t-T(\tau))\,d\tau$
$\displaystyle \rho(x,y,z,t)$	$\displaystyle =q\int_{-\infty}^\infty \frac{dT(\tau)}{d\tau} \delta(x-X(\tau))\delta(y-Y(\tau)) \delta(z-Z(\tau))\delta(t-T(\tau))\,d\tau$

a) Show that this current-charge density satisfies

$\displaystyle \nabla \cdot \vec J + \frac{\partial \rho}{\partial t}=0~.$

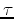Remark. The four-vector $\left(\frac{d\vec X(\tau)}{d\tau}, \frac{dT(\tau)}{d\tau} \right)$ is the charge's four-velocity in spacetime. The parameter $\tau$ is the ``wristwatch'' time (as measured by a comoving clock) attached to this charge.

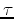b) By taking advantage of the fact $\frac{dT(\tau)}{d\tau}>0$ , evaluate the $\tau$ -integrals, and obtain explicit expressions for the components 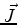$\vec J$ and $\rho$ .

Answer:

$\displaystyle \vec J(x,y,z,t)$ $\displaystyle = \,\frac{d\vec X}{dt}\,q\,\delta(x-X(t))\delta(y-Y(t))\delta(z-Z(t))\vec X(t)$ $\displaystyle =\vec X(\tau)\textrm{ evaluated at }\tau \textrm{ as determined by }\delta(t-T(\tau))~.$

$\displaystyle \rho(x,y,z,t)$	$\displaystyle =\quad\quad q\,\delta(x-X(t))\delta(y-Y(t))\delta(z-Z(t))$
where