Series and Convergence
So far we have learned about sequences of numbers. Now we will investigate
what may happen when we add all terms of a sequence together to form what will
be called an infinite series.
The old Greeks already wondered about this, and actually did not have the
tools to quite understand it This is illustrated by the old tale of Achilles and
the Tortoise.
Achilles, a fast runner, was asked to race against a tortoise. Achilles can run
10 meters per second, the tortoise only 5 meter per second. The track is 100
meters long. Achilles, being a fair sportsman, gives the tortoise 10 meter
advantage. Who will win ?
- Both start running, with the tortoise being 10 meters ahead.
- After one second, Achilles has reached the spot where the tortoise
started. The tortoise, in turn, has run 5 meters.
- Achilles runs again and reaches the spot the tortoise has just been. The
tortoise, in turn, has run 2.5 meters.
- Achilles runs again to the spot where the tortoise has just been. The
tortoise, in turn, has run another 1.25 meters ahead.
This continuous for a while, but whenever Achilles manages to reach the spot
where the tortoise has just been a split-second ago, the tortoise has again
covered a little bit of distance, and is still ahead of Achilles. Hence, as hard
as he tries, Achilles only manages to cut the remaining distance in half each
time, implying, of course, that Achilles can actually never reach the tortoise.
So, the tortoise wins the race, which does not make Achilles very happy at all.
Obviously, this is not true, but where is the mistake ?
Now let's return to mathematics. Before we can deal with any new objects, we
need to define them:
Definition: Series, Partial Sums, and Convergence
- Let { a n } be an infinite sequence.
- The formal expression
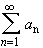is called an (infinite) series.
- For N = 1, 2, 3, ... the expression lim Sn =
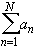is called the N-th partial sum of the series.
- If lim Sn exists and is finite, the series is said
to converge.
- If lim Sn does not exist or is infinite, the
series is said to diverge.
Note that while a series is the result of an infinite addition - which we do not
yet know how to handle - each partial sum is the sum of finitely many terms
only. Hence, the partial sums form a sequence, and we already know how to deal
with sequences.
Examples:
-
.gif)
= 1/2 + 1/4 + 1/8 + 1/16 + ... is an infinite series. The 3rd, 4th, and 5th
partial sums, for example, are, respectively: 0.875, 0.9375, and 0.96875.
-
Does this series converge or diverge ?
-
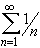= 1 + 1/2 + 1/3 + 1/4 + 1/5 + ... is another infinite series, called
harmonic series. The 3rd, 4th, and 5th partial sums are, respectively:
1.833, 2.0833, and 2.2833.
-
Does this series converge or diverge ?
Actually, if a series contains positive and negative terms, many of them may
cancel out when being added together. Hence, there are different modes of
convergence: one mode that applies to series with positive terms, and another
mode that applies to series whose terms may be negative and positive.
- A series
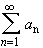converges absolutely if the sum of the absolute values
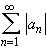converges.
- A series converges conditionally, if it converges, but not
absolutely.
-
Does the series
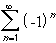converge absolutely, conditionally, or not at all ?
-
Does the series
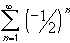converge absolutely, conditionally, or not at all ?
-
Does the series
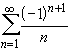converge absolutely, conditionally, or not at all (this series is called
alternating harmonic series) ?
Conditionally convergent sequences are rather difficult to work with. Several
operations that one would expect to be true do not hold for such series. The
perhaps most striking example is the associative law. Since a + b = b + a
for any two real numbers a and b, positive or negative, one would
expect also that changing the order of summation in a series should have little
effect on the outcome. However:
- Let
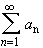be an absolutely convergent series. Then any rearrangement of terms in that
series results in a new series that is also absolutely convergent to the
same limit.
- Let
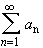be a conditionally convergent series. Then, for any real number c
there is a rearrangement of the series such that the new resulting series
will converge to c.
This will be proved as an exercise. One sees, however, that conditionally
convergent series probably contain a few surprises. Absolutely convergent
series, however, behave just as one would expect.
- Let
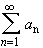and
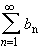be two absolutely convergent series. Then:
- The sum of the two series is again absolutely convergent. Its limit
is the sum of the limit of the two series.
- The difference of the two series is again absolutely convergent. Its
limit is the difference of the limit of the two series.
- The product of the two series is again absolutely convergent. Its
limit is the product of the limit of the two series (Cauchy
Product).
We will give one more rather abstract result on series before stating and
proving easy to use convergence criteria. The one result that is of more
theoretical importance is
- The series
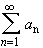converges if and only if for every
> 0 there is an integer N > 1 such that if n > m > N then
|
| <
|
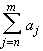