Open and Closed Sets
In the previous chapters we dealt with collections of points: sequences and
series. Each time, the collection of points was either finite or countable.and
the most important property of a point, in a sense, was its location in some
coordinate or number system. Now will deal with points, or more precisely with
sets of points, in a more abstract setting. The location of points relative to
each other will be more important than their absolute location, or size, in a
coordinate system. Therefore, concepts such as addition and multiplication will
not work anymore, and we will have to start, in a sense, at the beginning again.
All of the previous sections were, in effect, based on the natural numbers.
Those numbers were postulated as existing and all other properties - including
other number systems - were deduced from those numbers and a few principles of
logic.
We will now proceed in a similar way: first, we need to define the basic
objects we want to deal with, together with their most elementary properties.
Then we will develop a theory of those objects and called it topology.
- A set U
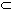 R is called open, if for each x
R is called open, if for each x
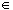 U there exists and
U there exists and
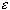 > 0 such that the interval ( x -
> 0 such that the interval ( x -
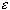 ,
x + ,
x +
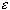 ) is contained in U. Such an interval is often called an
) is contained in U. Such an interval is often called an
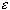 - neighborhood of x, or simply a neighborhood of x.
- neighborhood of x, or simply a neighborhood of x.
- A set F is called closed if the complement of F,
R \ F, is open.
- Which of the following sets are open, closed, both, or neither ?
-
The intervals (-3, 3), [4, 7], (-4, 5], (0,
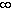 )
and [0, )
and [0,
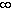 ) )
-
The sets R (the whole real line) and 0 (the empty set)
-
The set {1, 1/2, 1/3, 1/4, 1/5, ...} and {1, 1/2, 1/3, 1/4, ...}
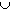 {0} {0}
It is fairly clear that when combining two open sets (either via union or
intersection) the resulting set is again open, and the same statement should be
true for closed sets. What about combining infinitely many sets ?
- Every union of open sets is again open.
- Every intersection of closed sets is again closed.
- Every finite intersection of open sets is again open
- Every finite union of closed sets is again closed.
How complicated can an open or closed set really be ? The basic open (or
closed) sets in the real line are the intervals, and they are certainly not
complicated. As it will turn out, open sets in the real line are generally easy,
while closed sets can be very complicated.
The worst-case scenario for the open sets, in fact, will be given in the next
result, and we will concentrate on closed sets for much of the rest of this
chapter.
- Let U
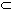 R be an arbitrary open set. Then there are countably many pairwise
disjoint open intervals
R be an arbitrary open set. Then there are countably many pairwise
disjoint open intervals
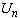 such that U =
such that U =
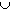
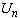
Next we need to establish some relationship between topology and our previous
studies, in particular sequences of real numbers. We shall need the following
definitions:
Definition: Boundary, Accumulation, Interior, and Isolated Points
- Let S be an arbitrary set in the real line R.
- A point b
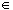 R is called boundary point of S if every non-empty
neighborhood of b intersects S and the complement of S.
The set of all boundary points of S is called the boundary of
S, denoted by bd(S).
R is called boundary point of S if every non-empty
neighborhood of b intersects S and the complement of S.
The set of all boundary points of S is called the boundary of
S, denoted by bd(S).
- A point s
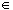 S
is called interior point of S if there exists a
neighborhood of S completely contained in S. The set of
all interior points of S is called the interior, denoted by
int(S). S
is called interior point of S if there exists a
neighborhood of S completely contained in S. The set of
all interior points of S is called the interior, denoted by
int(S).
- A point t
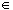 S
is called isolated point of S if there exists a
neighborhood U of t such that U S
is called isolated point of S if there exists a
neighborhood U of t such that U
 S
= {t}. S
= {t}.
- A point r
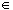 S
is called accumulation point, if every neighborhood of r contains
infinitely many distinct points of S. S
is called accumulation point, if every neighborhood of r contains
infinitely many distinct points of S.
-
What is the boundary and the interior of (0, 4), [-1, 2], R, and O
? Which points are isolated and accumulation points, if any ?
-
Find the boundary, interior, isolated and accumulation points, if any, for
the set {1, 1/2, 1/3, ... }
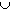 {0}
{0}
Here are some results that relate these various definitions with each other.
- Let S
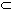 R. Then each point of S is either an interior point or a
boundary point.
R. Then each point of S is either an interior point or a
boundary point.
- Let S
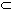 R. Then bd(S) = bd(R \ S).
R. Then bd(S) = bd(R \ S).
- A closed set contains all of its boundary points. An open set contains
none of its boundary points.
- Every non-isolated boundary point of a set S
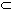 R is an accumulation point of S.
R is an accumulation point of S.
- An accumulation point is never an isolated point.
Finally, here is a theorem that relates these topological concepts with our
previous notion of sequences.
- A set S
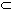 R is closed if and only if every Cauchy sequence of elements in S
has a limit that is contained in S.
R is closed if and only if every Cauchy sequence of elements in S
has a limit that is contained in S.
- Every bounded, infinite subset of R has an accumulation point.
- If S is closed and bounded, and
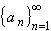 is any sequence in S, then there exists a subsequence
is any sequence in S, then there exists a subsequence
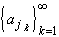 of
of
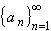 that converges to an element of S.
that converges to an element of S.
|

