Equations of Equilibrium
Consider a small cube of sides
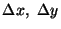and
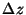with its centroid at point Q. Since different
faces of the cube do not pass through the same point, the state of stress on
them will be different. We assume that each component of stress is a
continuously differentiable function of
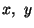and z. For a continuously differentiable function
f of x,
The components of stress on the six faces of the cube are shown
in Fig. 3.1
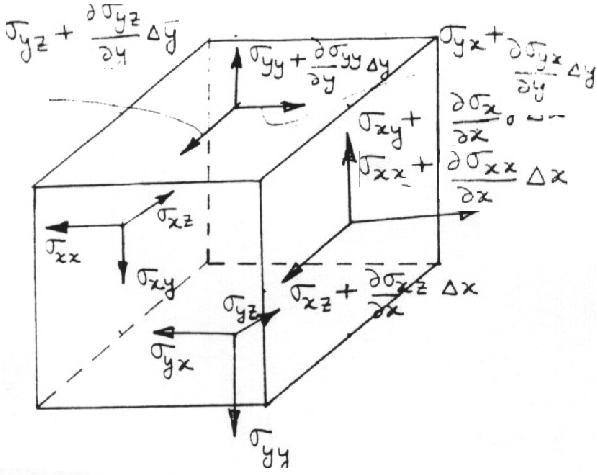
Let the gravitational force
g with components
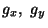 and
gz act at every point of the
cube;
g is measured as force/mass. The equilibrium of forces in
the x-direction gives
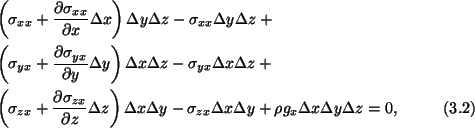
or
 |
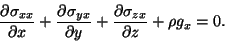(3.3) |
Similarly
 ,
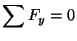and
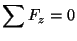give
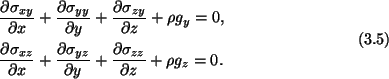
Here
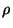is the mass density per unit volume of the body. Now take moments about the
z-axis passing through the centroid of the cube.
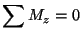gives
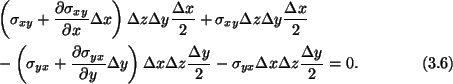
Divide by
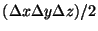to obtain
 |
(3.7) |
Let
and
 ,
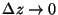and assume that
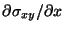and
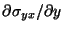are bounded. Equation (3.7) reduces to
 |
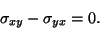(3.8) |
Similarly
Thus the balance of moments implies the symmetry of the stress components.
Results: Equations of Equilibrium:
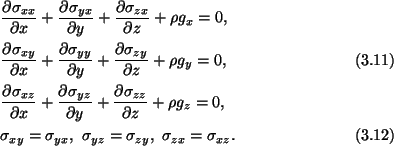
In condensed notation, we write
![\begin{displaymath}\{\sigma\}^T = [\sigma_{xx}\ \ \sigma_{yy}\ \ \sigma_{zz}\ \
\sigma_{yz}\ \ \sigma_{zx}\ \ \sigma_{xy}],\tag{3.13}
\end{displaymath}](/images/mechanical/equations/img41.gif) |
(3.13)) |
and in matrix notation
![\begin{displaymath}[{\mbox{\boldmath {$\sigma$ }}} ]= \left[\begin{array}{ccc}
\...
..._{zx} & \sigma_{zy} & \sigma_{zz}\end{array}\right].\tag{3.14}
\end{displaymath}](/images/mechanical/equations/img42.gif) |
(3.14) |
|
